Special Triangles (02:53)
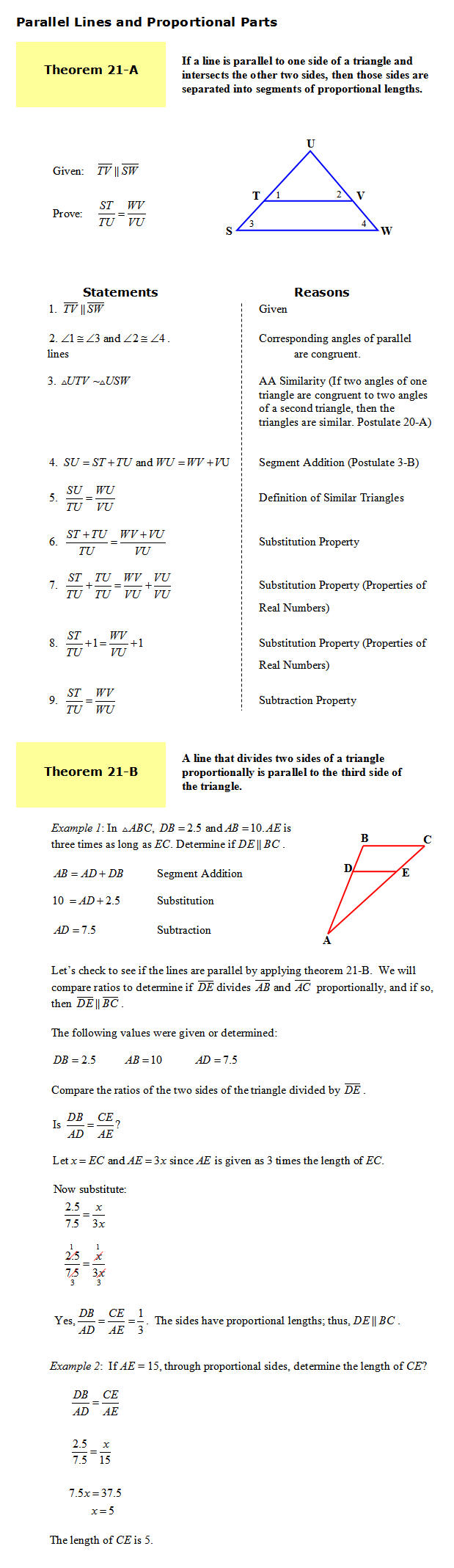
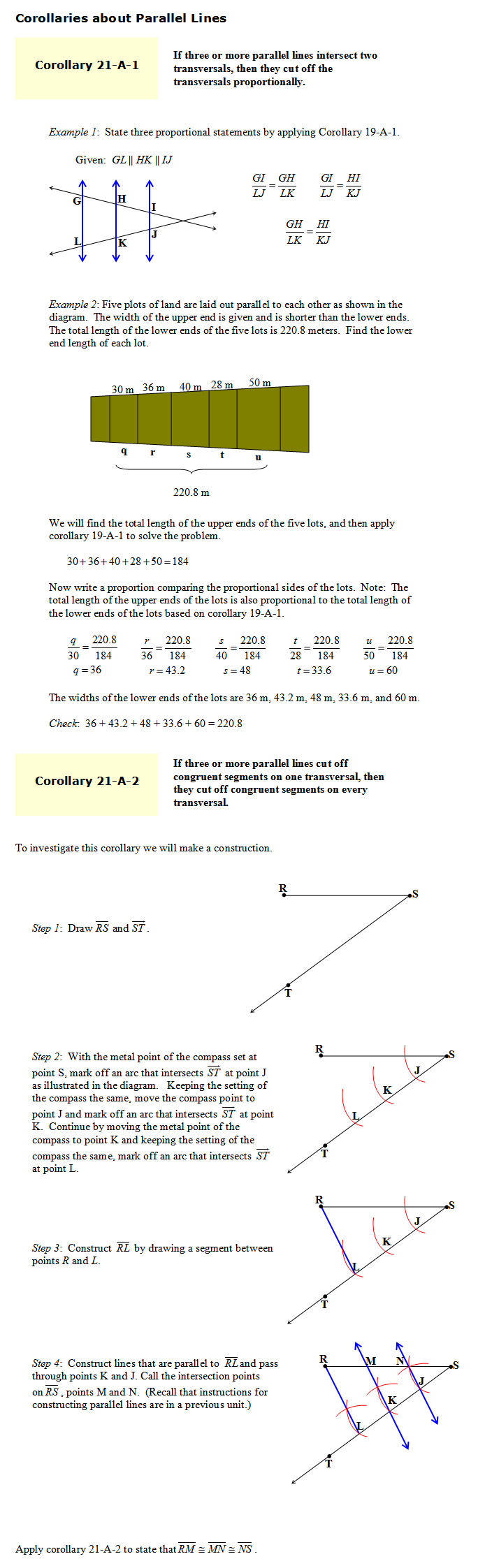
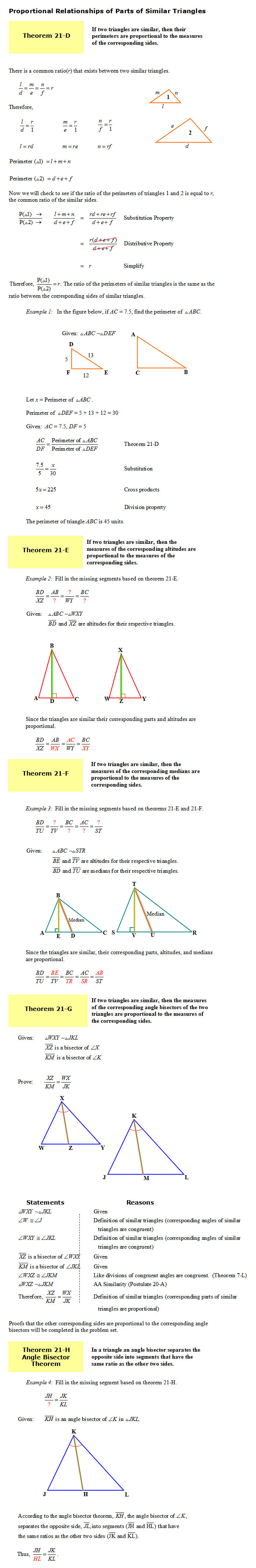
PROPORTIONAL PARTS OF SIMILAR TRIANGLES
In this unit, you will learn how parallel lines divide triangles into proportional parts, and that three or more parallel lines divide two transversals proportionally. You will examine the proportional relationships of similar triangles’ altitudes, medians, angle bisectors, and perimeters. You will use theorems, examples, and proofs to determine similarity.
|
| Special Triangles (02:53) |
|
|
|
 |
| Proportional Parts in Triangles and Parallel Lines |
| Midsegment of a Triangle |