INEQUALITIES AND ABSOLUTE VALUE EQUATIONS
Unit Overview
An inequality is a mathematical statement that compares algebraic expressions using greater than (>), less than (<), and other inequality symbols. A compound inequality is a pair of inequalities joined by and or or. In this unit, properties of inequalities will be used to solve linear inequalities and compound inequalities in one variable. The unit concludes continues with a study of absolute-value equations and inequalities.
Introduction to Solving Inequalities
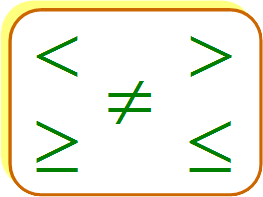
| Inequality - a mathematical statement that compares algebraic quantities |
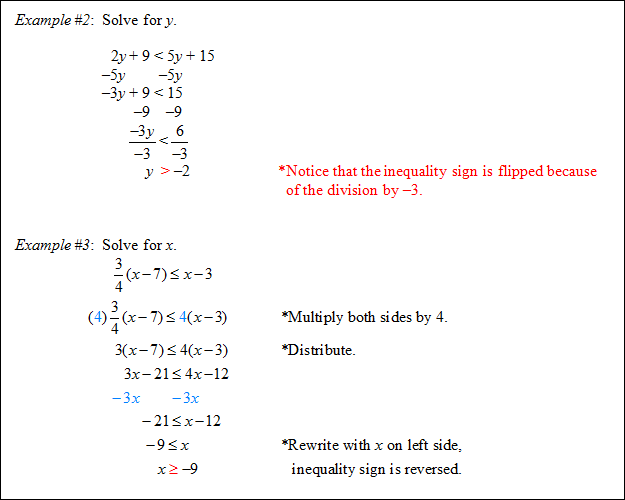
*Solving inequalities is just like solving equations, use opposite operations to isolate the variable.
 |
Inequalities--Bridge Capacity (02:27)
*When multiplying or dividing by a negative number, the inequality sign must be reversed.
 |
Solving Inequalities: Two Operations (01:25)
 |
You can represent the solution of an inequality in one variable on a number line.
For < and > an open circle is used to denote that the solution number is not included in the solution.
For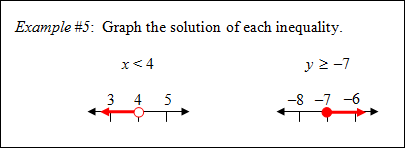
and 
a closed circle is used to denote that the solution number is included in the solution.  |
Compound Inequalities
| compound inequalities: a pair of inequalities joined by “and” or “or”. |
To solve a compound inequality joined with “and”, find the values of the variable that satisfy both inequalities.
| *“and” means the intersection of the solutions |
 |
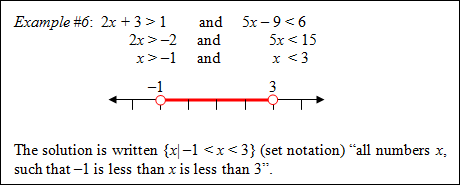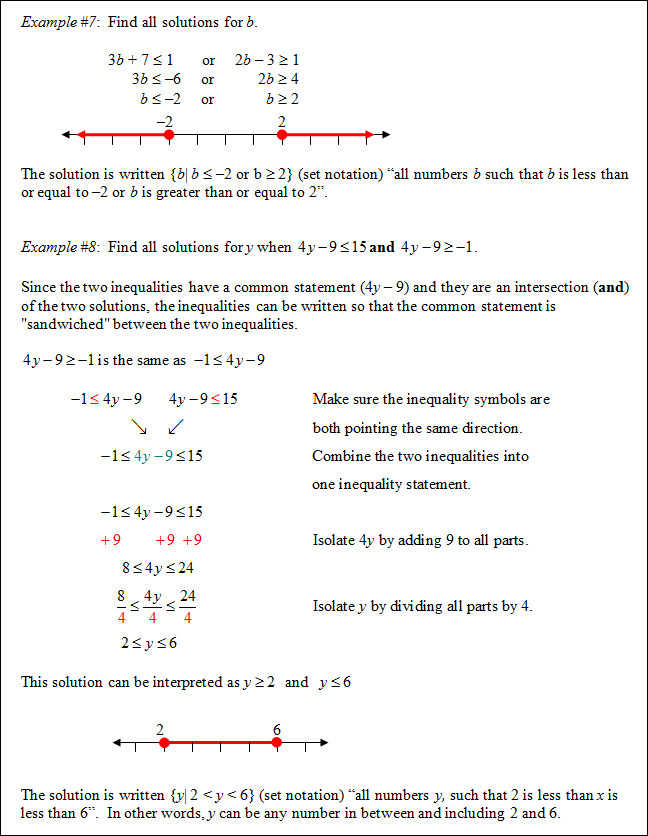
To solve a compound inequality joined with “or”, find the values of the variable that satisfy at least one inequality.
| “or” means the union of the solutions |
 |
Solving Compound Inequalities (02:25) 
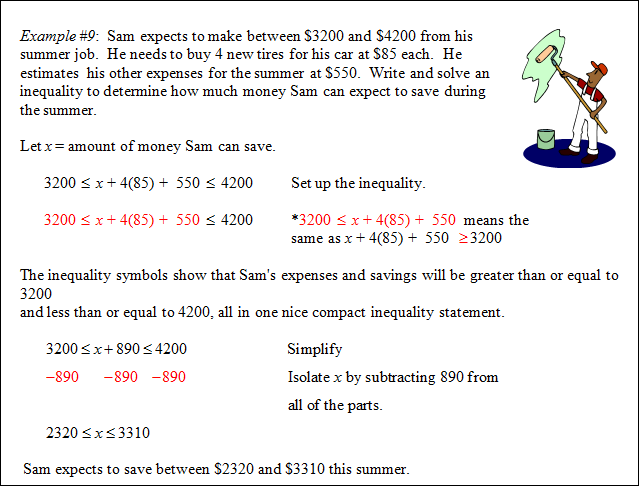
Stop! Go to Questions #1-13 about this section, then return to continue on to the next section.
Solving Absolute Value Equations and Inequalities
| absolute value - the distance a number is from zero (always positive). *Two bars around the number denote absolute value. |
= 5 
= 6Why do absolute value equations have two solutions?
In a simple absolute value equation such as | x | = 5, let's examine what two values of x make the expression true?
Since the | 5 | = 5, then x = 5.
Also, since the |–5 | = 5, then x = –5.
Therefore, the solution to | x | = 5 is x = 5 or x = –5.
Let's expand the understanding of an absolute value equation further.
Let's think about the following question: In the absolute value equation, |3x + 4| = 19, what two values can 3x + 4 be and why?
The value of 3x + 4 in |3x + 4| = 19 can be 19 because why?
The absolute value of |19| = 19, therefore 3x + 4 can equal 19.
"Click here" to check the answer.
The value of 3x + 4 in |3x + 4| = 19 can be –19 because why?
The absolute value of |–19| = 19, therefore 3x + 4 can equal –19.
"Click here" to check the answer.
What two equations can be written to find the values of x that solve |3x + 4| = 19?
3x + 4 = 19 OR 3x + 4 = –19
"Click here" to check the answer.
Follow these steps to solve absolute value equations, but keep in mind why these steps work by remembering the explanation above.
1.) Rewrite the equation without the absolute value notation.
2.) Rewrite a second time using the opposite of what the original equation was equal to, and connect with the word “or”.
3.) Solve both equations and check both answers in the original equation.
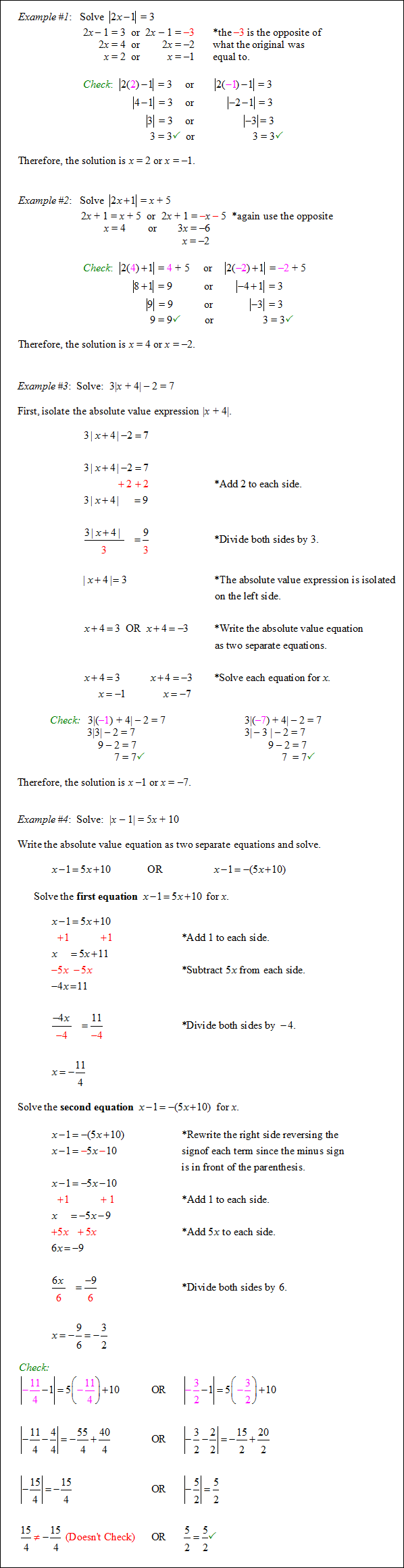
| extraneous solution: An extraneous is a solution that does not satisfy the original equation.
Since –11/4 does not satisfy the original equation, –11/4 is an extraneous solution. Therefore, the solution is x = –3/2. |
To solve absolute value inequalities:
1.) Rewrite the inequality without the absolute value notation.
2.) Rewrite a second time, change the inequality sign, and use opposites.
3.) Solve both inequalities and check both answers in the original inequality.
4.) If the inequality is a < or
5.) If the inequality is a > or
Stop! Go to Questions #14-33 to complete this unit.