INTEGERS AND EQUATIONS
 |
Unit Overview
In this unit, you will review integers and the rules that apply to adding, subtracting, multiplying and dividing these special numbers. You will also learn about equations and how the Real Number Properties of Equality justify the steps to solve an equation. You will also solve literal equations and formulas.
Comparing Integers
 |
 |
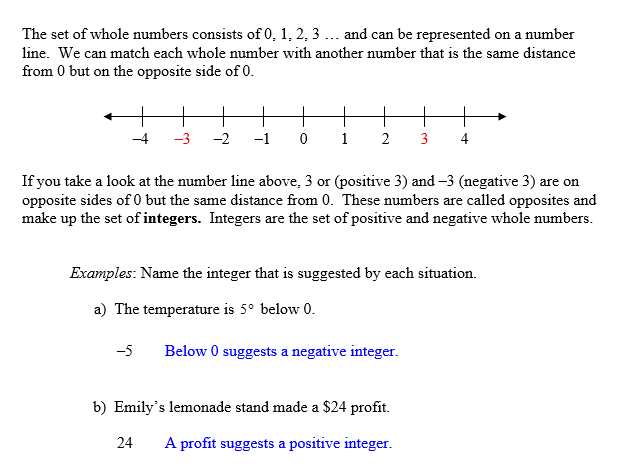Stop! Go to Questions #1-6 about this section, then return to continue on to the next section.
Adding and Subtracting Integers
 |
 |
Stop! Go to Questions #7-12 about this section, then return to continue on to the next section.
Multiplying and Dividing Integers
 |
Stop! Go to Questions #13-16 about this section, then return to continue on to the next section.
Real Number Properties of Equality
 |
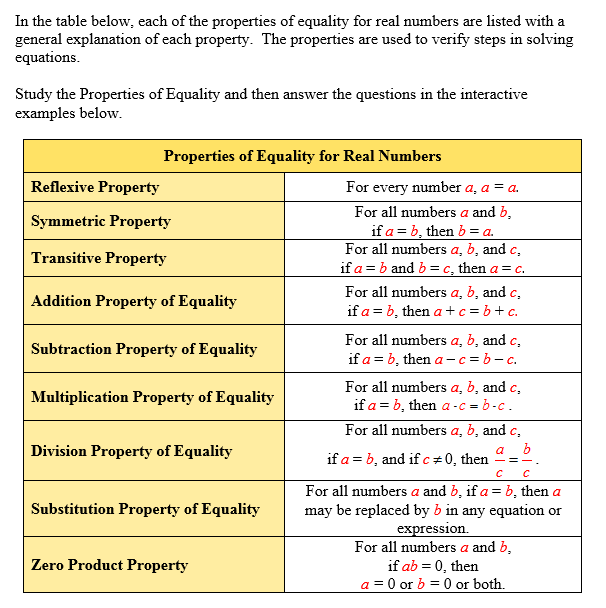| Examples: For each statement below, assume all variables represent real numbers. Identify each property illustrated by the statement. |
If x = y, then x + 7 = y + 7.
Addition Property of Equality
"Click here" to check the property.
If x = y and y = 8, then x = 8.
Transitive Property of Equality
"Click here" to check the property.
If x = y, then x(7) = y(7).
Multiplication Property of Equality
"Click here" to check the property.
15 = 15
Reflexive Property
"Click here" to check the property.
If x = y, then 
.
Division Property of Equality
"Click here" to check the property.
If x = 10, then 10 = x.
Symmetric Property of Equality
"Click here" to check the property.
If x = y + 4 and y = 6, then x = 6 + 4 = 10.
Substitution Property of Equality
"Click here" to check the property.
If x = y, then x – 7 = y – 7.
Subtraction Property of Equality
"Click here" to check the property.
If 9x = 0, then 9 = 0 (not true) or x = 0; thus, x = 0.
Zero Product Property
"Click here" to check the property.
The properties of real numbers will be very useful in solving equations algebraically.
Stop! Go to Questions #17-18 about this section, then return to continue on to the next section.
Solving Basic Equations
 |
The Golden X (15:06)  |
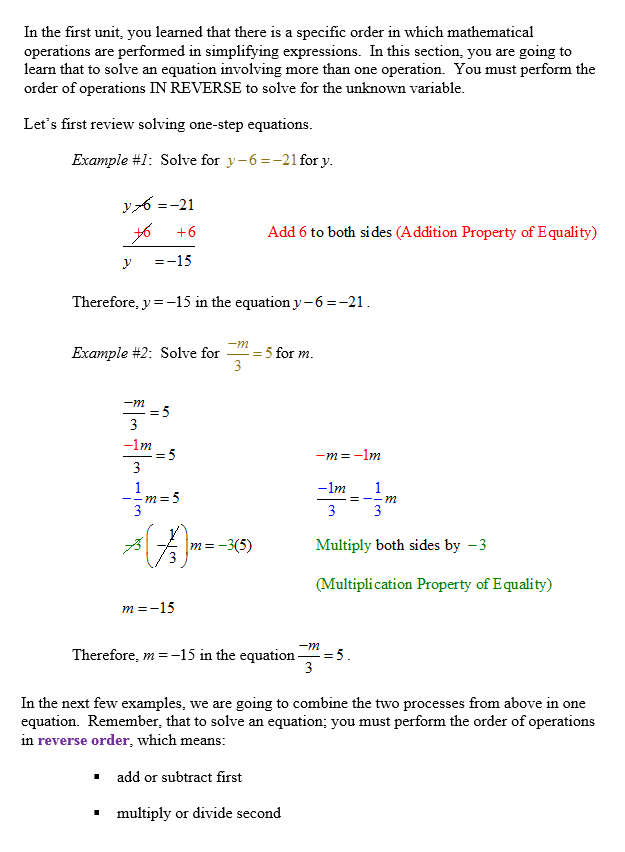Stop! Go to Questions #19-22 about this section, then return to continue on to the next section.
Literal Equations/Formulas
|
Now, let's solve the perimeter formula of a rectange for W.
| P = 2L + 2W |
What is the quantity of interest?
" W "
"Click here" to check the answer.
What inverse operation should be done first?
Subtract 2L from both sides.
"Click here" to check the answer.
What is the simplifed equation after performing the first inverse operation?
P – 2L = 2W
"Click here" to check the answer.
What inverse operation should be done next?
Divide both sides by 2.
"Click here" to check the answer.
What is the final equation of the perimeter formula solved for W ?
W = (P – 2L) / 2
"Click here" to check the answer.
 |
Stop! Go to Questions #23-24 about this section, then return to continue on to the next section.
Solving Equations with Variables on Both Sides
 |
|
 |
 |
 |
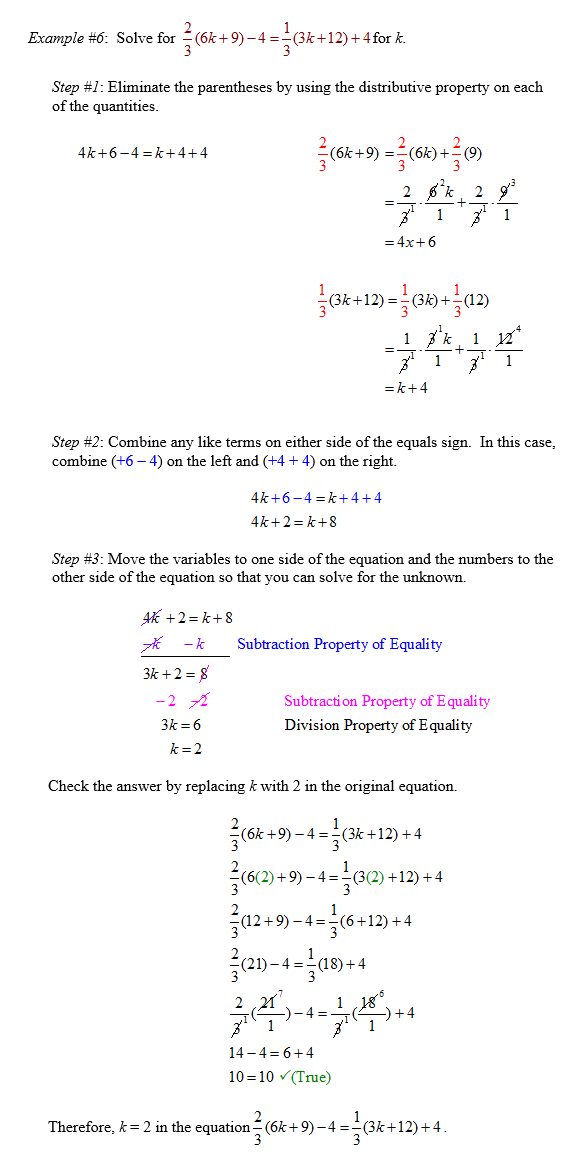Stop! Go to Questions #25-29 to complete this unit.
