Place Value and Expanded Notation
Each digit in a number has a place value. Let's look at the decimal number.
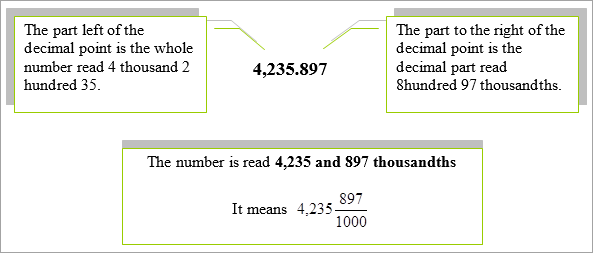
Now, let's look at the number in a place value chart.
DECIMALS: ADD, SUBTRACT, COMPARE, PLACE VALUE
Each digit in a number has a place value and we will look at place values in decimals to better understand the meaning of the decimal.
To compare decimals, write equivalent decimals with the same number of decimal places, then compare. Also, to put decimals in order, we first look at all the decimals we are ordering and then express them in the same number of decimal places.
To add or subtract decimals, we need to line up the decimal places? Why? That question is answered in the explanation of adding and subtracting decimals.
Writing equations and inequalities for word problems help us to understand the structure of a word problem. We’ll see how equations and inequalities can help us solve problems.
Place Value and Expanded Notation
Each digit in a number has a place value. Let's look at the decimal number.
|
 Decimal Numbers, Place Value |
| The Britannica login and password may be required for some of the activities. Login: vla Password: student |
Comparing Decimals
Example 1:
To compare decimals, write equivalent decimals with the same number of decimal places, then compare.
Compare 0.6 with 0.52
First write 0.6 as its equivalent decimal in hundredths, 0.60, then compare to 0.52.
0.6 is greater than 0.52 0.6 > 0.52
Example 2:
Here is another example of ordering decimals.
Put these decimals in order from least to greatest. 0.8, 0.25, 0.149
Since 0.149 is in thousandths and has three decimal places, rewrite the other two decimals in thousands also, filling in with zeros.
0.8 = 0.800 and 0.25 = 0.250
Now arrange them in order from least to greatest.
0.149 is less than 0.250 is less than 0.800
Now write the comparison using the original decimals.
0.149 < 0.25 < 0.8 |
| Comparing Decimal Numbers |
Add and Subtract Decimals
To add and subtract decimals, be sure to line up the decimal points so that the place values will also line up – tenths with tenths, hundredths with hundredths, and so on. Decimals may be expressed in equivalent fractions which help write the word name. Example 1: 7.2 = 7 2/10 = 7 and 2 tenths Example 2: 3.13 = 3 13/100 = 3 and 13 hundredths
|
| Add and Subtract Decimal Numbers |
| Decimal Numbers |
| Decimals and Fractions |
Adding and Subtracting Decimals |
Creating and Interpreting Equations and Inequalities
Cost equals price of one pencil plus a nickel for the second pencil.
Simplifying, the expression would be:
C = P + $0.05
Suppose the pencil that you chose cost $0.89 and you wanted a second one that was the same type. What would you pay for the two pencils on sale?
C = P + 0.05 C = 0.89 + 0.05 C= $0.94 for both pencils
If you gave the clerk $5.00 and there was no tax, write an expression that would represent the amount of change you would receive. Use G for change.
Write out the main points of the problem in words.
$0.94 plus the change would add up to $5.00
Simplifying, the expression would be:
$0.94 + G = $5.00
To find G, subtract. G = 5.00 - $0.94 G = $4.06
Let's look at another type of math statement, an inequality.
The price of the pencils in the store starts at $0.59. Which is the best description of the prices of the pencils?
a. Cost is less than $0.59 b. Cost is more than $0.59 c. Cost is greater than or equal to $0.59
Since the starting price is $0.59 and from there goes higher, the best description is c. Cost is greater than or equal to $0.59 |
 |
 Click on the icon to find and practice topics for this unit. |
| Unit 15 Compare Worksheet |
| Unit 15 Add and Subtract Decimals Worksheet |
The school store is having a sale. If you buy one pencil, you can buy a second pencil (