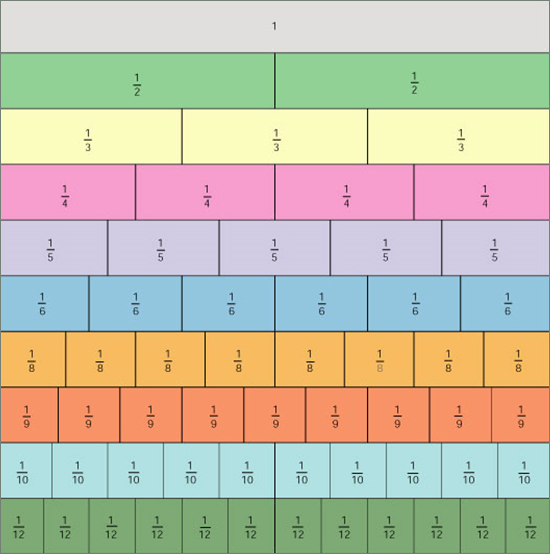
Fraction Bars
Look over these fraction strips. Each strip represents 1 whole.
1 = 2 halves, 3 thirds, 4 fourths, 5 fifths, 6 sixths, and so on.
Thus, 1 = 2/2 = 3/3 = 4/4 = 5/5 = 6/6 and so on…
SUBTRACTING MIXED FRACTIONS
To subtract fractions with unlike denominators, express the fractions into equivalent fractions with the same denominator. First we’ll look at models for subtracting mixed fractions using fraction bars and then we’ll examine the steps for solving subtraction problems without the fraction bars. We’ll also look at borrowing.
Write fraction answers using the form in these examples.
Example 1: two-thirds is written as 2/3.
Example 2: five and three fourths is written as 5 3/4.
Fraction Bars
Look over these fraction strips. Each strip represents 1 whole.
1 = 2 halves, 3 thirds, 4 fourths, 5 fifths, 6 sixths, and so on.
|
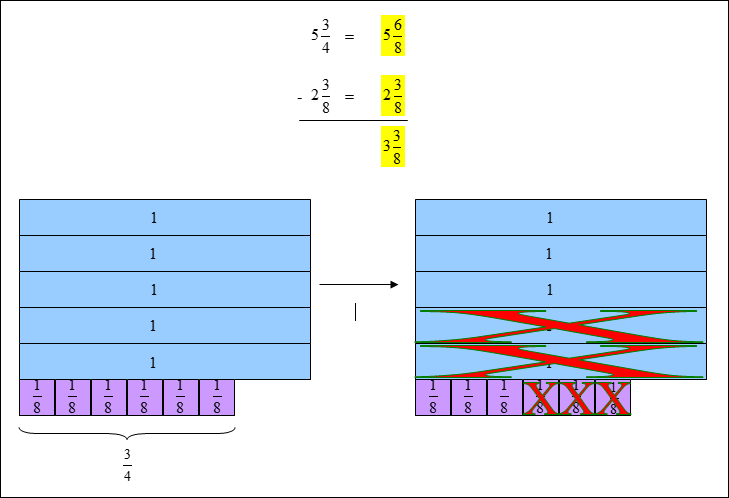
Subtracting Mixed Fractions Using Fraction Bars
Study the subtraction problem below. To subtract fractions with unlike denominators, express the fractions into equivalent fractions with the same denominator.
 |
Subtracting Mixed Fractions
Example 1: Find 8 14/15 – 2 3/5. Simplify, if necessary. |
Subtracting Mixed Fractions from Whole Numbers
Study the subtraction problem below. To subtract a mixed fraction from a whole number, express the whole number as 1 less. Rename the 1 as a fraction equivalent to one that has the same denominator as the mixed number. Example 1: Find 5 – 2 3/5. |
Subtracting Mixed Fractions and Borrowing Using Fraction Bars
Study the subtraction problem below. To subtract fractions with unlike denominators, first express the fractions into equivalent fractions with the same denominator. Then check to see if borrowing is needed to complete the problem.
|
Subtracting Mixed Fractions with Borrowing
|
 |
| Unit 11 Subtracting Mixed Numbers Worksheet |